Example. \(f(x)=3x^6-x^4+1.5x-1\) is a polynomial of degree 6. The coefficients of \(f\) are \(3,-1,1.5,-1\).
Domain of any polynomial function: \((-\infty,\infty)\).
Functions of the form \(f(x)=x^a\), where \(a\) is a constant.
Case 1. \(a=n\) is a positive integer


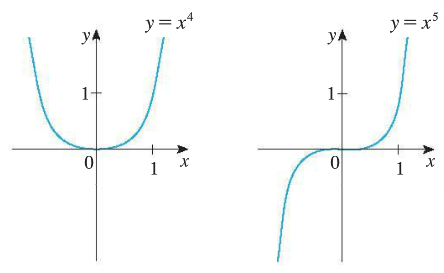
even power ==> even function, symmetric w.r.t. \(y\)-axis; range: \([0,\infty)\);
graphs of \(y=x^2,y=x^4,y=x^6,\dots\) look similar
odd power ==> odd function, symmetric w.r.t. origin;
range: \((-\infty,\infty)\);
graphs of \(y=x^3,y=x^5,y=x^7,\dots\) look similar
larger power ==> larger values of \(f(x)\) when \(x>1\)
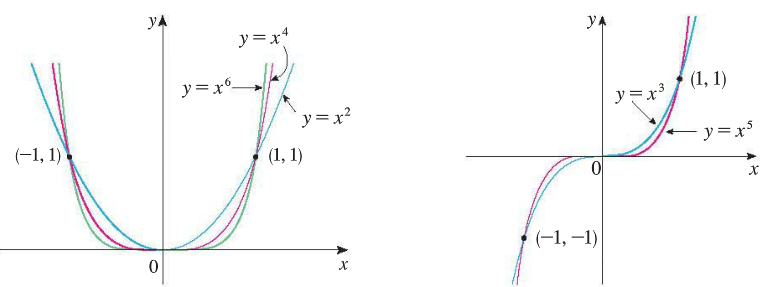
A simple trick to distinguish curves of different power functions:
for each function/graph, find the point when x=2, i.e., locate (2,f(2)).
larger value of f(2) ==> larger power.Case 2. \(a=1/n\) with \(n\) a positive integer
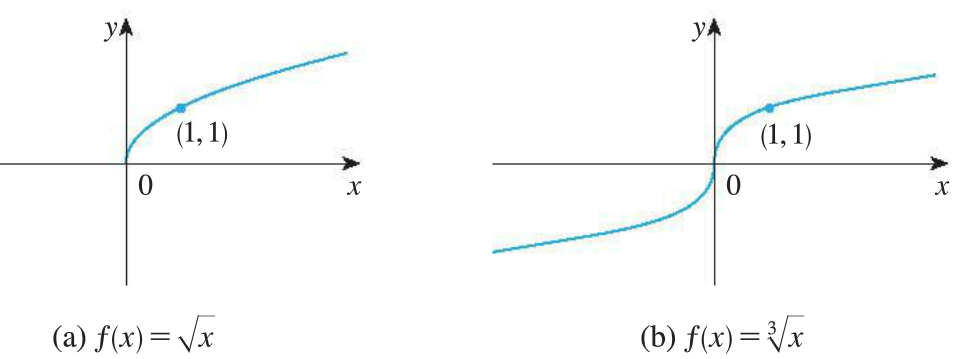
\(f(x)=x^{1/n}=\sqrt[n]{x}\)
even \(n\) ==> domain: \([0,\infty)\)
odd \(n\) ==> domain: \((-\infty,\infty)\)
For even \(n\) \((n= 2,4,6,\dots)\), graph of \(y=\sqrt[n]{x}\) is similar to that of \(y=\sqrt{x}\).
For odd \(n\) \((n= 3,5,7,\dots)\), graph of \(y=\sqrt[n]{x}\) is similar to that of \(y=\sqrt[3]{x}\).
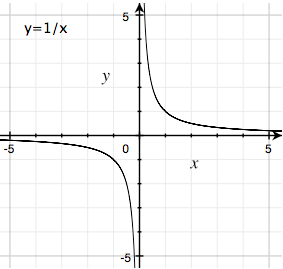
Case 3. \(a=-1\)
The reciprocal function \(f(x)=x^{-1}=1/x\) is an odd function. Graph of \(f\) is a hyperbola. Domain: \(x\neq 0\).
A rational function \(f\) is a ratio of polynomials: \(f(x)=p(x)/q(x)\) with polynomials \(p, q\).
The domain of \(f\) is the set of all \(x\) such that \(q(x)\neq 0\).
Examples. (a). \(f(x)=1/x\); (b). \(f(x)=(x^3+1)/(x^2-4)\).
(try to find their domains)
Algebraic functions: functions that can be constructed using algebraic operations (such as addition, subtraction, multiplication, division, and taking roots) starting with polynomails.
Simply speaking, algebraic functions are derived by mingling polynomials, power functions and rational functions.
Examples: (a). \(f(x)=\sqrt{x^2-1}\);
(b). \(g(x)= (x^4-5)/(x+\sqrt{x}) + (x^2-3)\sqrt{x+1}\).
(try to find their domains)
Convention in calculus: radian measure is used. That is, use \(\pi/2\) instead of \(90^{\circ}\).
See reviews on "Reference Page 2" and in "Appendix D" in the textbook.
For both sine and cosine functions, domain: \((-\infty,\infty)\); range: \([-1,1]\). \[-1\leq \sin x \leq 1\quad\quad -1\leq \cos x \leq 1\]
Periodic with a period of \(2\pi\): \(\quad \sin(x+2\pi)=\sin x\quad\quad \cos(x+2\pi)=\cos x\)
Tangent function: \(\tan x = \frac{\sin x}{\cos x}\)
More will be discussed in Section 1.4.
More will be discussed in Section 1.5.